Intersonic Motion of Mode I Cracks

PROJECT TITLE: INTERSONIC MOTION OF MODE I CRACKS

PARTICIPANTS: FABIAN BARRAS (PhD student at EPFL in Lausanne, Switzerland, and visiting scholar at Illinois), RENE CARPAIJ (MS student at EPFL and visiting scholar at Illinois), PROF. JEAN-FRANÇOIS MOLINARI (EPFL), AND PROF. PHILIPPE GEUBELLE


PROJECT DESCRIPTION: This project focuses on fundamental dynamic fracture problems involving a planar crack embedded in an infinite linearly elastic solid, subjected to tensile (pressure) loading, and interacting with a circular asperity. The objective is to investigate whether part of the deformed crack front can temporarily exceed the Rayleigh wave speed, which has long been shown to be the limiting speed for dynamically propagating mode I cracks. The analysis is performed using a spectral scheme (see references below), which allows for a very detailed description of the failure process taking place in the immediate vicinity of the propagating front. Simulations indicate that, for sufficiently strong asperities, the highly deformed front experiences a burst of inter sonic motion.

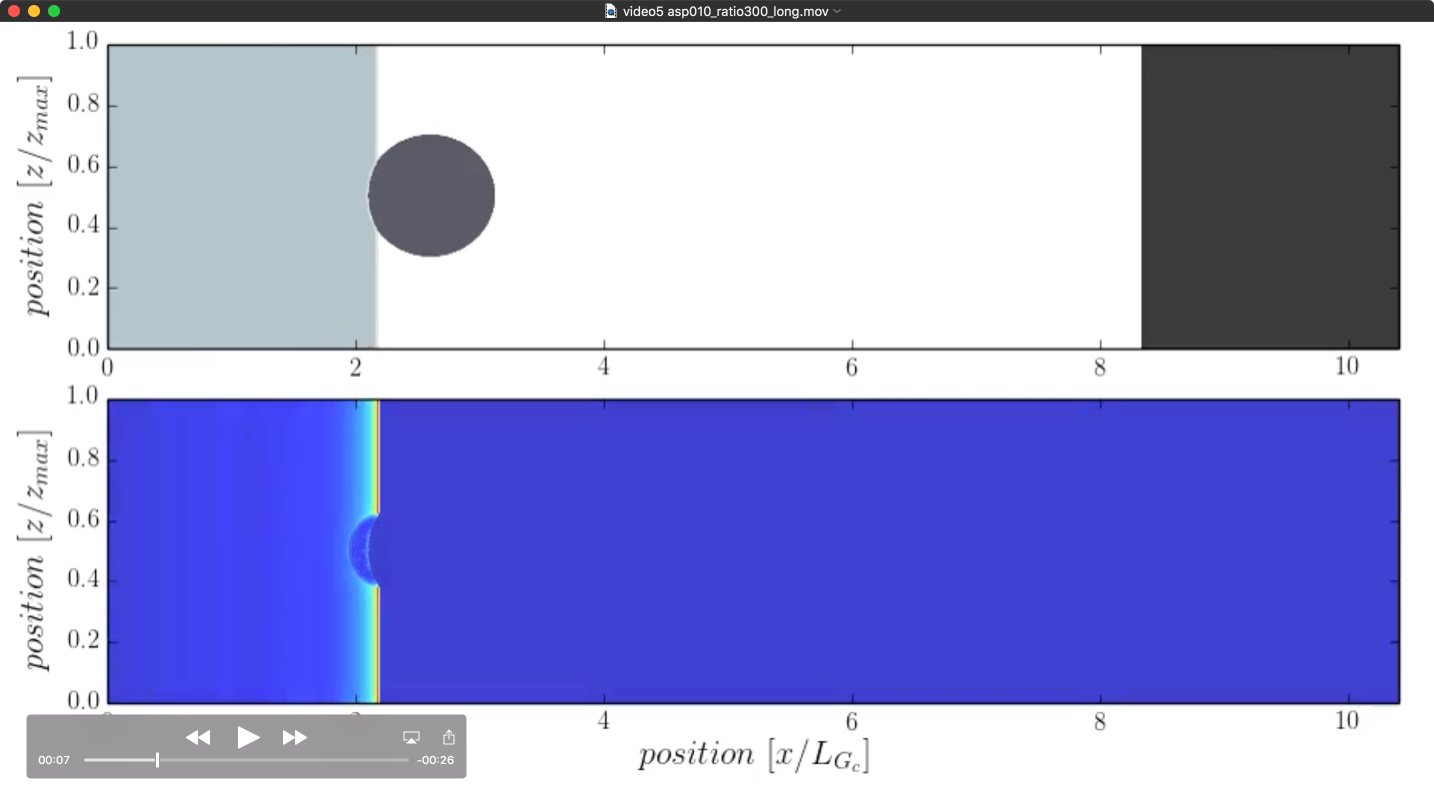
Top figure: Location of the planar crack (going from left to right) with an initially straight crack front at the time it starts to interact with the circular asperity (with a fracture toughness three times higher than its surrounding). The bottom figure shows the distribution of crack opening velocity.

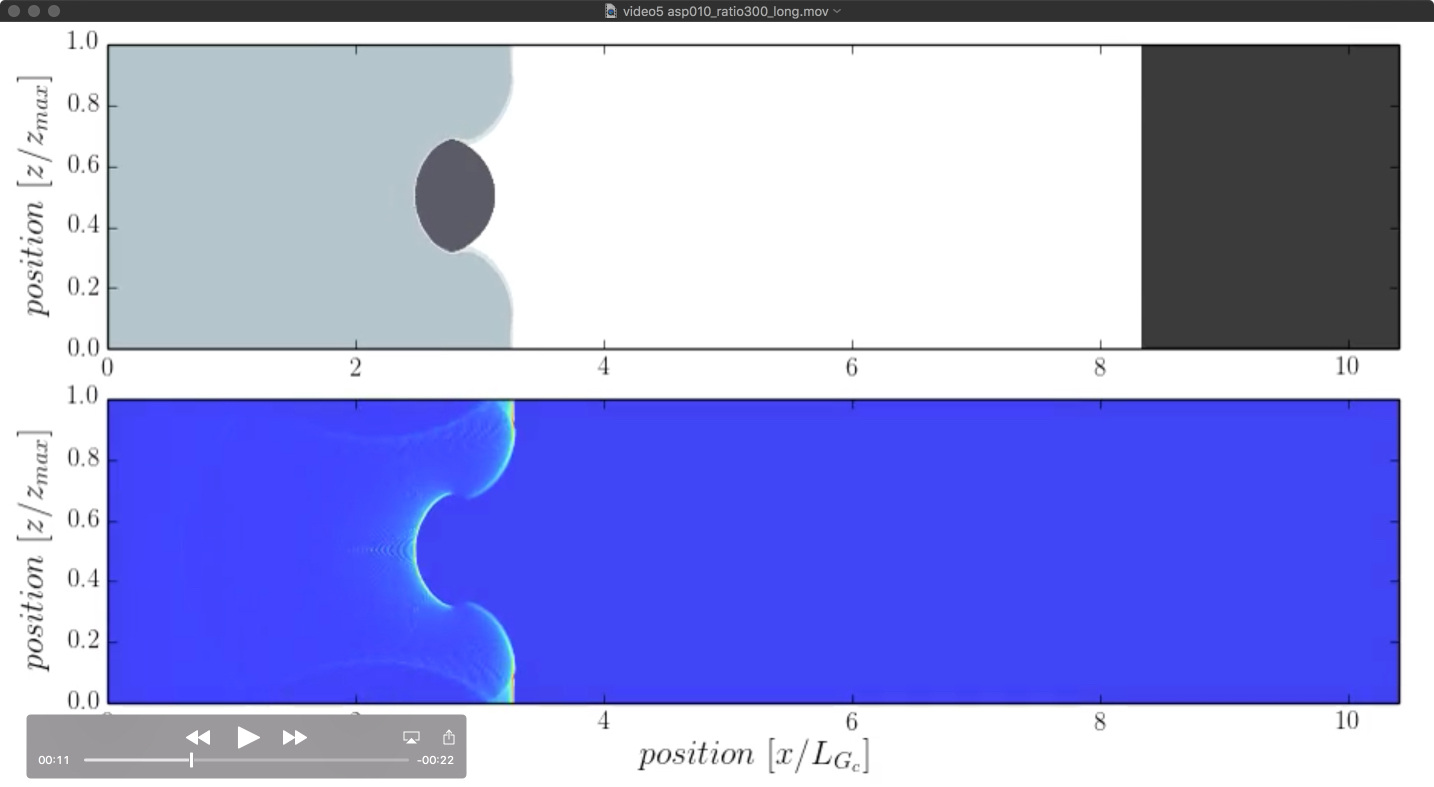
Deformation of the front, which starts to surround the failing asperity.

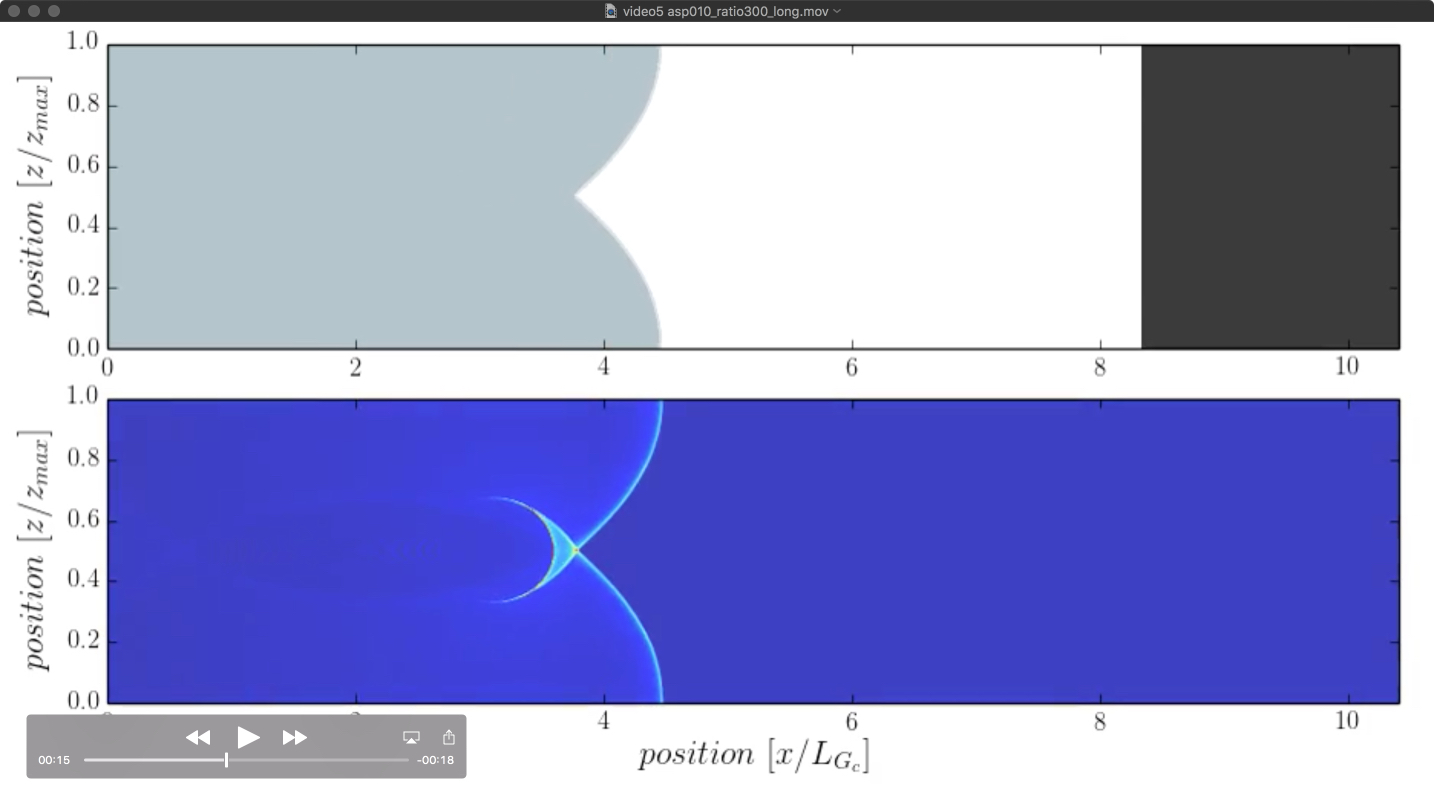
Crack front kink at the time of final failure of the asperity, showing the creation of a ‘Mach cone’, as the center of the crack propagates intersonically.

RELATED PUBLICATIONS:

  1. Geubelle, P. H. and Rice, J. R.  (1995) “A spectral method for 3D elastodynamic fracture problems”. J. Mech. Phys. Solids, 43:11, 1791-1824.
  2. Morrissey, J. W. and Geubelle, P. H.  (1997) “A numerical scheme for mode III dynamic fracture problems”. Int. J. Numer. Meth. Eng., 40, 1181-1196.
  3. Geubelle, P. H. and Breitenfeld, M. S.  (1997) “Numerical analysis of dynamic debonding under anti-plane shear loading”. Int. J. Fracture, 85, 265-282.
  4. Breitenfeld, M. S. and Geubelle, P. H.  (1998) “Numerical analysis of dynamic debonding under 2D in-plane and 3D loading”. Int. J. Fracture, 93, 13-38.
  5. Breitenfeld, M. S. and Geubelle, P. H.  (2000) “Parallel implementation of a spectral scheme for the simulation of 3D dynamic fracture events”.  Int. J. High Performance Computing Appl., 14:1, 26-38.